The outer Solar System in 5 billion years
Page 2 - Seeking answers about space? Join the Space community: the premier source of space exploration, innovation, and astronomy news, chronicling (and celebrating) humanity's ongoing expansion across the final frontier.
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
I was referring to radius, actually, so we agree.Helio, you are talking about the end of the red giant. I am talking about the (up to a ) billion years when it is much larger.
Cat
Oddly, I don't see how they got to those distances.Many thanks Helio, for those calculations.
Looking at #18, and this is a key whether or not it needs slight modification, it seems that at least Jupiter would be in the HZ. There is some variation, but the highest figure I have seen (above) for our Sun is 2AU diameter; thus 1 AU would have the Sun just reaching Earth (current) orbit.
If you bump the Sun to 1AU in radius, it will have a much larger surface area by a factor of about 46,200x what it is now. That's a whopping amount of radiation!
But, when it gets that large, it will be cooler. So if we use, say 3500K, then the ratio difference with the Sun is 0.61. The radiation, however, will be reduced by the 4th power of this difference, so eqch square meter of its surface will only be 13% as bright as the Sun's, but then multiply this by 46,500x more sq. meters than today's Sun.
This gives a luminosity that is 6,250x greater than today.
Since radiation decreases by the inverse square law, then we can simply take the square root of this to get the distance to match the effective radiation at 1 AU, which equates to 79. Meaning we would have to be at 79 AU to match what we have today.
The fact that at 3500K produces more in the IR likely will reduce that 79 AU but not in half, no doubt. So what am I missing?
I'm confident they aren't.Pogo, do we know whether these hot jupiters are mainly H and He?
Cat
Ok, now I'm not so confident. Sorry Pogo.I'm confident they aren't.
This paper addresses the effects of disassociation of hydrogen in ultra hot Jupiters. They don't mention escape velocity, however. Perhaps escape for H and He will be confined near the star, though I would assume stellar radiation and winds would eventually blow them away, too far to fall back to the planet. Interesting stuff!
Jupiter only has 2.5g for its gravity at the cloud tops, IIRC. So it's hard to imagine bumping those temperatures way up to high levels and not see a mass exodus off the planet.
Pogo, do we know whether these hot jupiters are mainly H and He?
See what you think of this. It addresses, I think, your atmospheric questions, but I haven't the time to look at it closely.
Thanks, Helio. The answer appears to be in Graph 3.
"Fig. 3: This graph shows gases that are prone to undergo Jeans’ escape on the basis of a ‘rule of thumb’ where Jeans’ escape is considered important if the mean thermal velocity of a gas at the exobase exceeds about a sixth of a planet’s escape speed. The temperature plotted for each celestial body corresponds to the average exobase temperature for objects with substantial atmospheres. For the Moon and Mercury, the average surface temperature is plotted. The sloping lines correspond to typical speeds of various gas molecules at the given temperature. For example, the graph shows that hydrogen and helium will easily undergo Jeans’ escape on Mars because the lines for these gases lie above the one-sixth escape speed marked by the dot for Mars, whereas water, oxygen, nitrogen and carbon dioxide will not undergo Jean’s escape because the lines for these gases lie below the threshold for Mars. Similarly, we see that hydrogen will not escape from the giant planets" My emphasis.
Cat
"Fig. 3: This graph shows gases that are prone to undergo Jeans’ escape on the basis of a ‘rule of thumb’ where Jeans’ escape is considered important if the mean thermal velocity of a gas at the exobase exceeds about a sixth of a planet’s escape speed. The temperature plotted for each celestial body corresponds to the average exobase temperature for objects with substantial atmospheres. For the Moon and Mercury, the average surface temperature is plotted. The sloping lines correspond to typical speeds of various gas molecules at the given temperature. For example, the graph shows that hydrogen and helium will easily undergo Jeans’ escape on Mars because the lines for these gases lie above the one-sixth escape speed marked by the dot for Mars, whereas water, oxygen, nitrogen and carbon dioxide will not undergo Jean’s escape because the lines for these gases lie below the threshold for Mars. Similarly, we see that hydrogen will not escape from the giant planets" My emphasis.
Cat
I see that "hot jupiters" (small j) may be unlike our Jupiter, in that they they do not necessarily depend so heavy on H and He. How important do you think this might be?
Cat
Cat
Yeah, not sure what the atmospheres are, just remember reading about them. I guess I just assumed they were H/He. I’ll have to look around. Maybe they just hadn’t been close in long enough to burn off the atmosphere. I defer to Helio’s expertise.
Given high surface temperatures, a lot of compounds will become a gas, thus these would add to them atmospher.I see that "hot jupiters" (small j) may be unlike our Jupiter, in that they they do not necessarily depend so heavy on H and He. How important do you think this might be?
But isn’t the large mass of Jupiter due heavily to H & He? Is so, then if a lot of the lighter elements escape, then perhaps it would have less gravity, causing more escaping gas.
Helio, yes, I understand H and He should dominate statistically, but there are suggestions of more exotic atmospheres. I also understand that the Sun consists mainly of H and He, that it is very hot, and that it is surrounded by a (good) partial vacuum - yet it retains its H/He because of gravity. So Jupiter is no longer verging on becoming a star, but the same principles must apply - making due allowance for Jupiter's lower gravity. I think Fig. 3 gives some clues.
Cat
Cat
Yep. But its escape velocity is more than 10x that of Jupiter.Helio, yes, I understand H and He should dominate statistically, but there are suggestions of more exotic atmospheres. I also understand that the Sun consists mainly of H and He, that it is very hot, and that it is surrounded by a (good) partial vacuum - yet it retains its H/He because of gravity.
Yep, gravity is the only thing holding it and Fig. 3 does a nice job comparing the planet's values.So Jupiter is no longer verging on becoming a star, but the same principles must apply - making due allowance for Jupiter's lower gravity. I think Fig. 3 gives some clues.
Now that you've referenced this, I see that only 1/6th the escape velocity is needed for escape. This is more than I would have guessed, but as you know better than I do, and volume of gas has a wide range of individual velocities, so if the temperature is enough to reach 1/6th escape velocity, they are saying that there will be some particles (e.g. H) that will be faster than the actual escape velocity. Since billions of years is part of the picture, then a steady escaping at an average of 1/6th value makes sense.
Helio, if we check it out, I guess that will probably show up in a distribution curve.
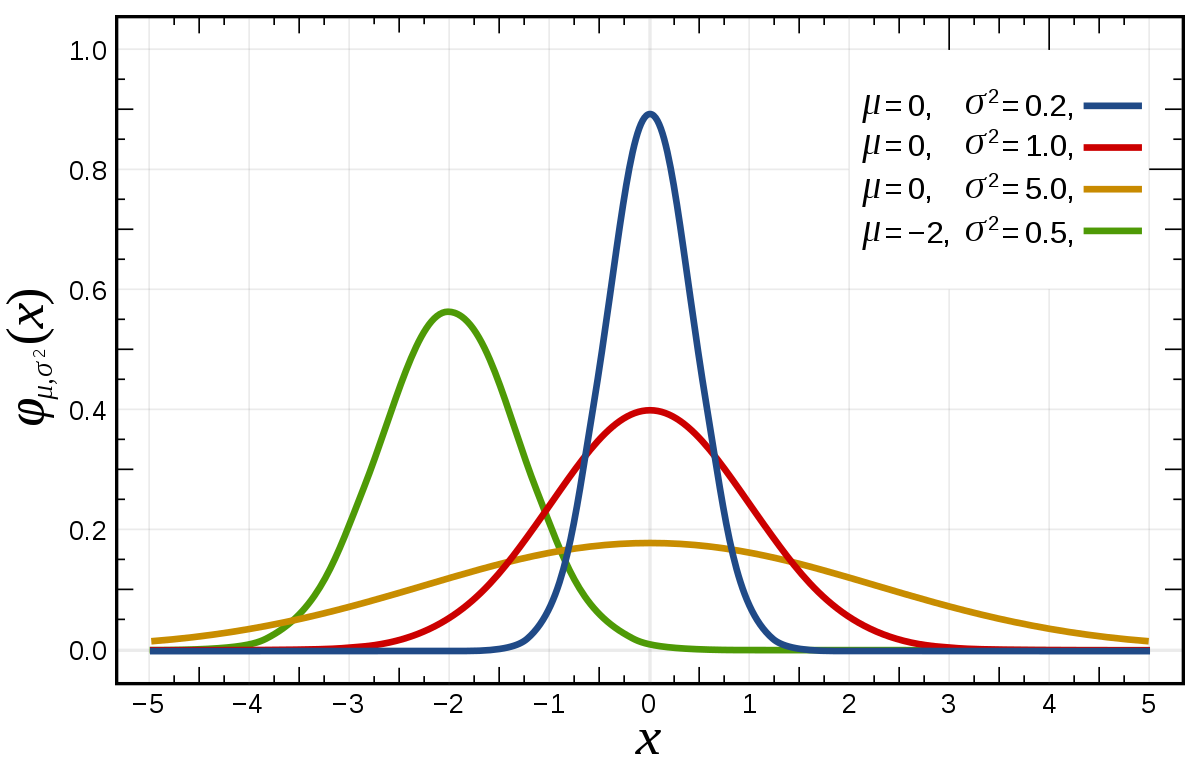
 en.wikipedia.org
en.wikipedia.org
View: https://imgur.com/a/uGci3gt
Looks like 1/6 is where the normal curve gets steep - or is it just my coloured spectacles?
Cat

Normal distribution - Wikipedia
 en.wikipedia.org
en.wikipedia.org
View: https://imgur.com/a/uGci3gt
Looks like 1/6 is where the normal curve gets steep - or is it just my coloured spectacles?
Cat
Last edited:
Can you convert that curve to Texan?Helio, if we check it out, I guess that will probably show up in a distribution curve.

Normal distribution - Wikipedia
en.wikipedia.org
View: https://imgur.com/a/uGci3gt
Looks like 1/6 is where the normal curve gets steep - or is it just my coloured spectacles?
y is the number/proportion of people with property x. So few (y) have the smallest or largest property, most have the average, or most common property.
So the expected escape velocity x (mid point) has the most number of particles. Few (low y) have values much smaller or much larger than the escape velocity.
If you look at the bottom graph (cumulative) Few have exceptionally low values. More and more get values approaching the average/expected and then there is little/no increase in the number of those getting more than the expected.
Cat
So the expected escape velocity x (mid point) has the most number of particles. Few (low y) have values much smaller or much larger than the escape velocity.
If you look at the bottom graph (cumulative) Few have exceptionally low values. More and more get values approaching the average/expected and then there is little/no increase in the number of those getting more than the expected.
Cat
Last edited:
Ok,
I found one showing the distribution of velocities of certain gases here. H would be spread much greater in velocity since it is 1/2 the mass (H2).
I found one showing the distribution of velocities of certain gases here. H would be spread much greater in velocity since it is 1/2 the mass (H2).
Thank you Helio.
There is an explanation here: https://www.scribd.com/document/455587330/Maxwell-Boltzmann-distribution
You may need Ctrl = or similar to enlarge it. [or maybe not, apparently]
Here is the Wiki version:
Maxwell–Boltzmann distribution - Wikipedia
Same explanation as #40. Vertical axis is number of particles, and x axis is proportion with that number.
Cat
There is an explanation here: https://www.scribd.com/document/455587330/Maxwell-Boltzmann-distribution
You may need Ctrl = or similar to enlarge it. [or maybe not, apparently]
Here is the Wiki version:
Maxwell–Boltzmann distribution - Wikipedia
Same explanation as #40. Vertical axis is number of particles, and x axis is proportion with that number.
Cat
Last edited:
Similar threads
- Replies
- 11
- Views
- 2K
- Replies
- 0
- Views
- 597
- Replies
- 14
- Views
- 7K
TRENDING THREADS
-
New interpretation of QM, with new two-phase cosmology, solves 15 foundational problems in one go.
- Started by Geoff Dann
- Replies: 320
-
Hubble Tension explained (including its value) by the two phase cosmology
- Started by Geoff Dann
- Replies: 210
-
Basic Error: The accelerating Universe conclusion - reason
- Started by Gibsense
- Replies: 263
-
-
The birth of the Quantum Convergence Threshold (QCT):
- Started by Capanda Research
- Replies: 84
-
-

Space.com is part of Future plc, an international media group and leading digital publisher. Visit our corporate site.
© Future Publishing Limited Quay House, The Ambury, Bath BA1 1UA. All rights reserved. England and Wales company registration number 2008885.

